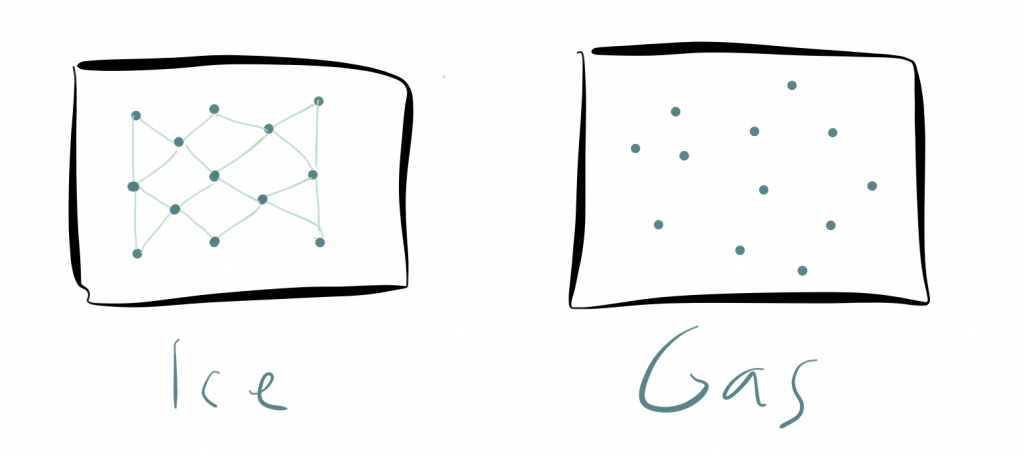This is part 2 of my mini-series on understanding symmetry breaking, Goldstone’s theorem and the Higgs mechanism intuitively. Part 1 is here.
The punchline of the Higgs mechanism is often summarized as:
For example, in the standard model, we break the $SU(2)$ gauge symmetry. Since gauge transformations depend on the location $G=G(x)$ they are local and therefore no Goldstone bosons appear when we break it.
Unfortunately, such a summary of the Higgs mechanism has many problems and leads to a lot of confusions.
The problems all have to do with the following observation: Before we can calculate anything that we can compare with experiments, we must remove the gauge symmetry by fixing the gauge. Usually, in the textbooks, the Higgs mechanism is discussed before the gauge has been fixed. Then, there is no obvious problem. We discuss breaking of the gauge symmetry and then fix the gauge to remove the gauge symmetry completely.
However, what happens if we reverse these two steps? We can first fix the gauge and then have a look what the Higgs mechanism is doing. Certainly, then we can’t talk about breaking of the gauge symmetry since it has been removed completely from the theory. What is the Higgs mechanism then doing and why are there no Goldstone bosons? The story gets even weirder because there are different possible ways to fix a gauge. The story of what the Higgs mechanism is doing changes depending on how we fix the gauge. We can even remove the $SU(2)$ symmetry completely by changing the field variables (See: J. Fröhlich, G. Morchio and F. Strocchi, Phys. Lett. B97, 249 (1980) and Nucl. Phys. B190, 553-582 (1981)).
If all this weren’t bad enough there is even a famous theorem, called Elitzur’s theorem, whose punchline is:
I don’t want to dive into the details here, but if you want to have a look at what people are discussing in this context have a look at this paper.
The same confusing situation does not only exist in particle physics. The Higgs mechanism is often invoked to explain how superconductors work. Analogous to the story in particle physics, students are usually taught that here the electromagnetic $U(1)$ gauge symmetry is broken. The cooper pairs play then the role of the Higgs field and since the broken $U(1)$ symmetry is local no Goldstone bosons appear in the spectrum. (See, for example, the discussion in An Invitation to Quantum Field Theory by Luis Alvarez-Gaumé and Miguel A. Vázquez-Mozo). Again, we run into lots of difficulties if we have a closer look as discussed, for example, in this article.
Now the good news.
Since we already understand symmetry breaking and Goldstone’s theorem intuitively, it is not that hard to understand how the Higgs mechanism works. Especially, we will not run into confusing situations as the ones outlined above, since we will stick to physical things.
Much of the confusion surrounding the Higgs mechanism can be attributed to the confusion surrounding gauge symmetries. I will discuss gauge symmetries in another post since to understand the Higgs mechanism it is sufficient to stick to what is physical about gauge symmetries and leave all the mysticism aside.
The loophole in Goldstone’s theorem
Like for most theorem in physics, there are loopholes in Goldstone’s theorem. Especially, there are systems where the configuration with the lowest energy, the ground state, breaks a symmetry, but no Goldstone modes exist.
To spoil the surprise: in these systems, no Goldstone modes exist because there are long-range forces present before the symmetry breaks. Such long-range forces are what is physical about gauge symmetries and this explains the connection between gauge symmetries and the Higgs mechanism.
The simplest example is again a ferromagnet. As mentioned above, usually the spins of the individual atoms only talk to their nearest neighbors. In other words, there are no long-range forces. Below the Curie temperature, the spins align, but it costs no energy to perform a rotation of all spins at once. Such a uniform rotation is a “spin wave” with infinite wavelength and thus our Goldstone mode here. It appears here because the fundamental laws are rotational invariant and only the ground state of the ferromagnet below the Curie temperature, i.e. the configuration with all spins aligned, breaks the symmetry.
However, if there is additionally a long-range force present in the system, for example, the $1/r$ Coulomb force, such a global uniform rotation costs energy, because we must work against the Coulomb force. Therefore, as soon as long-range forces are present there are no Goldstone modes.
Instead, what happens is that the long-range force becomes short-ranges as soon as the phase transition happens (in the example above, below the Curie temperature). The long-range force waves combine with the would-be Goldstone modes and the result is a short-range force. The waves with an infinite wavelength that would be the Goldstone modes now also have an effect on the long-range force, e.g. on the electric field. Thus such a wave with infinite wavelength is no longer possible without costing energy.
Instead, in the case of the ferromagnet, when we consider such a global uniform rotation what we get is a charge-density wave. This charge density wave is independent of the wavelength. As a result, the Goldstone modes have a finite non-zero frequency.
As mentioned above in part 1, symmetries break when the system becomes rigid. Below the Curie temperature, the ferromagnet resists rotations of individual spins. As a result of this rigidity, the former long-range force becomes short ranged.
The long-range electromagnetic force is mediated by electromagnetic waves, which simply means oscillations of electric and magnetic fields. When the system has become rigid, these electromagnetic waves can no longer propagate freely. The displacement of individual spins and thus of individual magnetic moments cost energy if the system is rigid. Hence, the system tries to minimize such displacements by intruding magnetic oscillations. As a result, the electromagnetic waves get damped and thus no longer have an infinite range.
In particle physics terms, we say the photon now has a mass. Before the symmetry breaking the photon is massless which means the range of electromagnetic interactions is infinite. After the system has become rigid the range of electromagnetic interactions is finite and this means the particle mediating the interaction is massive
The Higgs mechanism in particle physics
“Anatoly Larkin, posed a challenge to two outstanding undergraduate teenage theorists, Sacha Polyakov and Sasha Migdal: “In field theory the vacuum is like a substance; what happens there?”
from Chapter 9 in The Infinity Puzzle, by F. Close
As already mentioned in the introduction above there is a folklore that is repeated over and over in the textbooks. According to the folklore the Higgs mechanism exploits a loophole in Goldstone’s theorem because the symmetry that gets broken is a local one. This is wrong. A local symmetry is not a symmetry, but merely a redundancy in the description and cannot be broken anyway.
The real loophole, as discussed above, is that we consider a system with long-range forces. Prior to the phase transition into a ground state with smaller global symmetry, we have massless spin $1$ bosons that mediate the long-range forces. The scalar field then undergoes a phase transition and condenses into a new rigid ground state. This new ground state does no longer correspond to an empty vacuum, but to a uniform distribution of Higgs field, which could be called “Higgs Substance”, to borrow a phrase from Guidice’s “A Zeptospace Odyssey“.
In particle physics, the conventional formulation of the non-empty vacuum state is that we say that the Higgs has a non-zero vacuum expectation value.
- A non-zero vacuum expectation value means that on average we expect to see some Higgs excitations in the vacuum, i.e. the vacuum is filled with Higgs field excitations.
- A zero vacuum expectation value means that we see on average no Higgs excitations if we observe the vacuum, i.e. the vacuum is empty.
The point is that below some critical temperature the configuration with the lowest energy is no longer an empty state, but one that is filled with the Higgs substance. The spontaneous filling of the vacuum with the Higgs substance is completely analogous to how a ferromagnet becomes filled with magnetization below the Curie temperature. In this sense, the vacuum is not empty but rather more like a medium.
The spontaneous alignment of the spins in a ferromagnet picks randomly a direction in space and breaks, therefore, rotational symmetry. Analogously, the Higgs field picks a direction in the internal $SU(2) \times U(1)$ space. Before the vacuum becomes filled with the Higgs substance there is no way to distinguish the three $SU(2)$ bosons. Only after the Higgs spontaneously picks a direction, these bosons become distinguishable.
There is an important second effect. Special relativity tells us that massless particles move with the speed of light while massive particles always move slower. A direct consequence of a vacuum filled with Higgs substance is that all particles that interact with this substance can no longer move freely. Instead, whenever they try to get from A to B, they are stopped all the time by the Higgs substance. Hence, they no longer move with the maximum velocity, i.e. the speed of light. In this sense they acquire an effective mass through the permanent interaction with the Higgs substance. However, the Higgs substance makes its presence not only felt when particles are moving.
Instead, the permanent interaction with the Higgs substance also happens when particles are at rest. Without the Higgs substance filled vacuum, all particles would move with the speed of light.
To summarize: The real loophole that makes the Higgs mechanism possible are long-range interactions. Whenever we are dealing with a system with long-range interactions, there are no Goldstone bosons after symmetry breaking, i.e. when the system becomes rigid. After symmetry breaking, the long-range interaction becomes short-ranged and in particle physics terms this means that the corresponding boson is now no longer massless but massive. An important second effect is that other formerly massless field excitations can become massive through the now rigid structure. In particle physics particles interact all the time with the “Higgs substance” that fills all of the vacuum after symmetry breaking.
To read more about what the Higgs mechanism is really doing in more abstract terms have a look at this post, especially the last section.